In the late nineteenth and early twentieth centuries, a great deal of experimental evidence began to accumulate for which classical mechanics could provide no explanation. It was the consideration and explanation of these data which led to the development of quantum mechanics.
One of the most significant failures of classical mechanics was its inability to explain the distribution of energy emitted by a black body. Any hot object emits electromagnetic radiation, and the maximum in the emitted wavelength shifts to shorter wavelengths as the temperature of the emitter is raised.
A black body is the name given to a theoretical ideal emitter, an object capable of absorbing and emitting all wavelengths of radiation equally. A black body emitter may be successfully approximated by a small opening into a heated cavity. The emission curves of a black body have the following form:
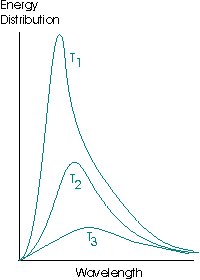
The energy distribution, or spectral energy density, is the energy per unit volume of the cavity that is emitted in the wavelength interval λ to λ + dλ. Note the total area under the curve increases as the temperature increases, indicating that the hotter an object is, the more energy it radiates per unit volume.where T1 > T2 > T3.
Analysis of the data from black body emitters led to the formulation of the Wien displacement law relating the temperature and the wavelength of maximum energy density:
![]()
The problem of the energy distribution may be treated classically by considering the electromagnetic field as a collection of oscillators of all possible frequencies. The presence of radiation of frequency ν then signifies that the oscillator of that frequency has been excited. The classical equipartition principle may then be applied to determine the average energy of each independent oscillator. From this basis follows an expression known as the Rayleigh-Jeans law after the physicists who originally formulated it:
![]()
where ρ is the spectral energy density.
This law is very successful at long wavelengths but fails badly at short ones – the inverse dependence upon λ means that as the wavelength gets shorter and shorter, the spectral energy density tends to infinity. This result is patently absurd, as it suggests that even at room temperature, objects should radiate strongly in the high frequency portion of the spectrum (gamma rays, x-rays and the ultraviolet), which is clearly not the case. This failing of the law is termed the ultraviolet catastrophe:
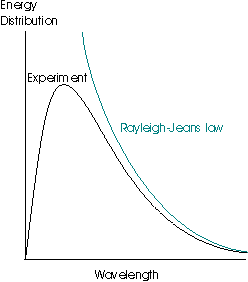
This idea was originally proposed by the physicist Planck, and he discovered that the observed data were reproduced if he supposed that the energies of an oscillator of frequency ν were limited to integer multiples of hν, where h was a fundamental constant which is now known as the Planck constant. This assumption allowed him to derive the Planck distribution: The experimental observations may be accounted for by limiting the energy of each electromagnetic oscillator to discrete values. (This is quite contrary to the classical view, in which all possible energy values are allowed.) The name given to this limitation of possible values is quantisation of energy.
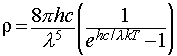
with ρ the spectral energy density.
This expression provides a good agreement with experimental data, and the constant h, which is an undetermined parameter in the original theory, may be adjusted to obtain the best fit. This allows measurement of the value of h by experiment.
This expression is similar in form to the Rayleigh-Jeans law, the main difference being in the exponential term in the denominator. At short wavelengths the exponential term is large, and as the wavelength tends to zero, the exponential term tends to infinity faster than the λ5 term tends to zero. The upshot of this is that as the wavelength tends to zero, so does the spectral energy density. Thus the ultraviolet catastrophe is avoided.
At long wavelengths, the Planck distribution reduces to the Rayleigh-Jeans law.
This is because the exponential term in the distribution is much smaller than one when the wavelength is large, so it may be approximated by 1 + (hc / λkT). (Recall that ex ≈ 1+ x when x is very small.) Substitution of this approximation into the Planck distribution immediately gives the Rayleigh-Jeans law.
The Wien law may also be obtained from the Planck distribution. In this case we are looking for the position of the maximum in the distribution. To find this we set dρ/dλ = 0 , and make the approximation that the wavelength is so short that hc/λ>>kT. This gives
![]()
and when the values of the fundamental constants are substituted in, the Wien law is obtained.
The reasoning behind the success of the Planck distribution is as follows:
Rayleigh’s approach failed because it assumed that the thermal motion of atoms in the walls of a black body would excite all the electromagnetic oscillators equally; the ultraviolet catastrophe is a result of the excitation of high frequency oscillators.
According to Planck’s hypothesis, however, an oscillator cannot be excited unless it receives an energy of at least hν (as this the minimum amount of energy an oscillator of frequency ν may possess above zero. It cannot have an amount of energy which is a fraction of hν, so it cannot accept an amount of energy less than hν). For high frequency oscillators (large ν), the amount of energy hν is too large to be supplied by the thermal motion of the atoms in the walls, and so they are not excited.
Quantisation of energy reduces the contribution to the emission curve of high frequency oscillators, as the energy available is not sufficient to excite them.