Atomic and Molecular Spectra
Some of the most impressive evidence for non-classical behaviour comes from the spectra of atoms and molecules. Rather than showing emission or absorption of radiation at all frequencies, nonzero intensities are only observed at discrete frequency values:
 |
This diagram is a schematic representation of a typical atomic emission spectrum. Note the discrete lines, rather than a smooth curve which would indicate emission occurring at all frequencies. |
The simplest explanation for this is to invoke quantisation of energy, the entirely non-classical idea that the energy of an atom or molecule is confined to certain discrete values, and that all other energy values are not permitted. (Energy quantisation was first introduced to solve the problem of the radiation profile of a black body emitter.)
If only certain energy values are permitted, then energy can only be lost or gained in amounts that are equal to the difference in energy between two of the permitted values. These energy differences are each represented by a line in the spectrum, through the relationship ΔE = hν.
Heat Capacities
Early in the nineteenth century, the Dulong-Petit law was proposed, which states that:
The molar heat capacity of all monatomic solids is the same, and approximately equal to 25 J K-1 mol-1.
This law was based purely upon empirical observations, however it is very easy to justify in terms of classical physics. Classically, the equipartition principle states that the mean energy of an atom as it oscillates about a mean position in a solid is kT for each direction in which it may be displaced. Since an atom can oscillate in the x, y and z directions, it follows that the average energy of each atom in the solid is 3kT. We can therefore write the following expression for the molar internal energy of a monatomic solid:
![]()
We may then use the definition of the constant volume heat capacity to write:
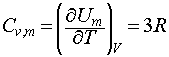
which, since 3R = 24.9 J K-1 mol-1 , is in good agreement with the Dulong-Petit law.
However, when it became possible to measure heat capacities at lower temperatures, significant deviations from the law were observed. The values were lower than 3R, and it was realised that as T tends to zero, so does the heat capacity.
The explanation of this again lies in the quantisation of energy. The atoms oscillate around their mean position with a frequency ν, and the energy of each of these oscillators is quantised into integral multiples of hν. At high temperatures, kT>hν, and so all the oscillators are active. The solid then has its classical heat capacity value.
However, at lower temperatures, the thermal energy kT may be smaller than hν if ν is large. This means that at lower temperatures, higher frequency oscillators cannot draw enough energy from the surroundings to become excited. Thus they do not contribute to the heat capacity, and its value is lower than the classically calculated value. At T = 0 , none of the oscillators have any energy, so none of them are excited, and the heat capacity of the solid is thus zero.