In this model, we consider a particle that is confined to a rectangular plane, of length L_x in the x direction and L_y in the y direction. The potential energy is zero everywhere in this plane, and infinite at its walls and beyond. It should be clear that this is an extension of the particle in a one-dimensional box to two dimensions.

The wavefunction is now a function of both x and y, and the Schrodinger equation for the system is thus:

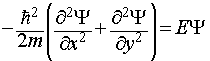
This is a partial differential equation, involving more than one variable (x and y). However, the form of this equation is such that it proves possible to separate it into two ordinary differential equations, one for each variable.

We start by making the assumption (which turns out to be true in this case) that the wavefunction Ψ can be written as a product of two functions, X(x), which depends solely on x, and Y(y), which depends solely upon y. i.e. Ψ(x,y) = X(x)Y(y). Substitution of this expression and manipulation of the resulting equation allows us to deduce the following two expressions.

where E_x is the energy associated with the particle's motion in the x direction, and E_y is the energy associated with the particle's motion in the y direction. This implies that the total energy, E, is the sum of E_x and E_y.

Each of these two ordinary differential equations is the same as the Schrodinger equation for the particle in a one-dimensional box, so we may immediately write down the solutions:

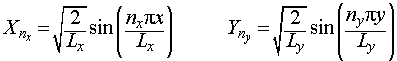
Since we have defined Ψ = XY and E = E_x + E_y, we may now write the overall wavefunction for a particle in a 2-D box as:

where 0 £ x £ L_x and 0 £ y £ L_y. The total energy is given by:

The quantum numbers n_x and n_y can independently take any positive integral value.

Note that this type of treatment may be extended to a particle in a three dimensional box in precisely the same way. The equation obtained for the wavefunction has an extra factor for the z dependence, and the equation for the energy has an extra term, also for the z dependence.

A situation that requires consideration is when the plane surface the particle is confined to is square. i.e. the situation where L_x = L_y = L. In this instance, the above equations for the wavefunction and the energy become:

By inspection of these two equations, it should be possible to see that when n_x = 1 and n_y = 2 the wavefunction is different from the situation in which n_x = 2 and n_y = 1. However, the energies of the system are the same in both situations ( 5h²/8mL²).

When two different wavefunctions correspond to the same energy, the condition is known as degeneracy.

In this case, the energy level 5h²/8mL² is said to be doubly degenerate. Note that degeneracy occurs when there is high symmetry in the system, i.e. when the plane the particle is confined to is square, but not when the sides are of unequal length.