The rotational constant of a vibrationally excited state of a diatomic molecule will be slightly smaller than that of the vibrational ground state, as the excited state will have a slightly longer bond than the ground state (due to the anharmonicity of the vibration). Consequently the moment of inertia of the excited state will be greater and its rotational constant will be smaller.
This is why the Q-branch of a spectrum (if there is one) consists of a series of closely spaced lines. It also means that the lines of the R-branch converge slightly with increasing J and the lines of the P-branch diverge slightly with increasing J. The actual expressions for the transitions (with B0 the rotational constant of the ground state and B1 the rotational constant of the first excited state) are:
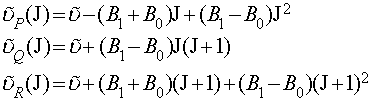
The method of combination differences is used to determine the two rotational constants. This is essentially a form of manipulation of simultaneous equations, where we choose two transitions to or from a common state, write out the equations for these transitions and then eliminate the rotational constant for the common state. i.e. the R-branch transition from J-1 to J and the P-branch transition from J+1 to J have a common final state (J). It is simple, from the above relations, to prove the following equation:
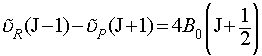
i.e. we have been able to use the fact that these two transitions have a common final level to remove the rotational constant of that level from the equation. Determination of B0 is now trivial from experimental data.