The heading of this page refers to the hypothetical system that will be considered on it. Though in itself this problem may appear quite a trivial one, it introduces various important concepts, and paves the way for exploration of some slightly more complex and physically relevant systems. It may also itself be used as a first approximation to some actual physical problems.
A particle in a one-dimensional box is the name given to a hypothetical situation where a particle of mass m is confined between two walls, at x=0 and x=L. In the infinite square well that we will consider, the potential energy is zero within the box but rises instantaneously to infinity at the walls. Note that a particle cannot be found in a region where the potential is infinite, as the particle would then have infinite energy:
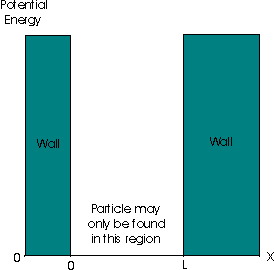
Within the box, the Schrodinger equation for the particle is precisely the same as that for a particle in free space ( V = 0), which was solved on this page. We may thus immediately write out the wavefunction for the particle within the box as:
However, it will prove more convenient to use the basic relation eiθ = cosθ + isinθ to write the solution in this form:
where C and D are two more arbitrary constants, and 0 £ x £ L .
Now, because of the presence of the potential walls, we shall see that further restrictions upon the permitted wavefunctions arise. For x > L or x < 0 , the wavefunction may be simply be stated to be Ψ = 0 , since the particle may not be found in these regions.
We have established that for the total wavefunction of the particle to be acceptable it must be continuous. The wavefunction for the particle within the box must be zero at the walls for it to be continuous with the wavefunction for the particle within the walls. This imposes two boundary conditions upon the wavefunction Ψk:
![]()
(The notation ψk(x) means the value of the wavefunction Ψk at the point x.)
If we use the first of the boundary conditions we obtain 0 = C (as cos 0 = 1 and sin 0 = 1) which implies that the wavefunction for the particle in a one-dimensional box reduces to
If we then put x = L and apply the second boundary condition, we obtain:
![]()
There are two ways this equation may be satisfied. First, D may be equal to zero. However, this means that the wavefunction for the particle would be zero everywhere, which means the particle does not exist. Such a solution, though mathematically acceptable, is ruled out on physical grounds.
The boundary condition may thus only be satisfied if kL is an integer multiple of π, as the sine of any integer multiple of pi is zero. Thus we may write:
![]()
Note that n = 0 is not an acceptable solution, as it implies k = 0, which again makes the wavefunction zero everywhere. The acceptable wavefunctions are therefore:
and n is a positive integer.
Note that the above equation tells us that the energy of the particle is now quantised, limited to discrete values. This quantisation arises due to the restriction of n to discrete values, and this arises out of a need to fulfil the boundary conditions imposed on the system.
It is a general observation that quantisation of a physical property such as the energy arises due to boundary conditions, as it is these conditions that render some solutions unacceptable.
Note also that the gaps between adjacent energy levels decrease as the integer n increases.
We shall now normalise the wave function. Since it is real (does not contain i) its square modulus is the same as the square of the wavefunction. We integrate this over all space available to the particle and require the integral to be equal to one (since the particle is certain to be found somewhere in the space that it is physically permitted to occupy). Note that since the particle may not be found in regions of infinite potential, the limits on the integration are 0 and L :
This integral may be solved using standard integration techniques, which allow us to obtain the answer C = (2/L)½
Thus the complete, normalised wavefunction for the particle in a one-dimensional box is:
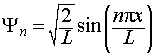
The wavefunctions and energies are labeled with a quantum number, n.
A quantum number is a number (an integer, or in some cases a half-integer) which labels the state of the system. For a particle in a box, there are an infinite number of acceptable wavefunctions (and thus an infinite numbers of states in which the system may found) , and the quantum number n specifies which state the system is in.
| The energy and wavefunction of a given state often depend solely upon the quantum number of that state. This diagram represents the first three wavefunctions of a particle in a 1-D box, each labeled with their quantum number, n: |
These wavefunctions are all sine functions of the same amplitude but with different wavelengths. Note that the degree of curvature of the waves increases with n. Since curvature of a wavefunction is directly related to the kinetic energy implied by the wavefunction, we can say this indicates that the energy of the particle increases with n also. This can be confirmed from inspection of the equation for the energy of the particle.
The fact that n cannot be zero means that the lowest amount of energy the particle may possess (when n is 1) is not zero, as would be permitted in classical mechanics (and would imply a completely stationary particle) but is in fact:
This minimum, irremovable energy of the particle is called its zero-point energy.
The existence of a zero-point energy may be explained two ways:
Firstly, we may consider the wavefunctions that satisfy the boundary conditions of a particle in a box. The wavefunction must be zero at both walls of the box, and smooth, continuous and nonzero elsewhere. This implies that a satisfactory wavefunction must be curved, and curvature of a wavefunction automatically implies the possession of kinetic energy.
Secondly, the situation may be considered in terms of the uncertainty principle (see here for a full explanation of the principle). Roughly speaking, the principle asserts that the uncertainty in the position of a particle multiplied by the uncertainty in its linear momentum must be greater than or equal to / 2 .
Now, if the particle had zero energy, it would have no kinetic energy, and so would have to be stationary. Its linear momentum would then have a precisely defined value of zero. Since the uncertainty in the linear momentum is zero, the only way for the uncertainty principle not to be violated is for the uncertainty in the particle’s position to be infinite. However, for a particle in a box this can never occur – the uncertainty in its position can never be infinite as we know it must be somewhere between the two walls that define the box. Thus it is not possible for a particle in a box to be stationary, and hence it must possess a zero-point energy.
The final property of a particle in a box that needs to be examined is the probability density, which according to the Born interpretation is given by:
Note that in a case such as this, where the wavefunction is purely real, the square modulus of the wavefunction is equivalent to the square of the function.
This probability density varies with position within the box. Below the first two wavefunctions for a particle in a box are shown, and below them are corresponding probability densities, as defined by the above equation:
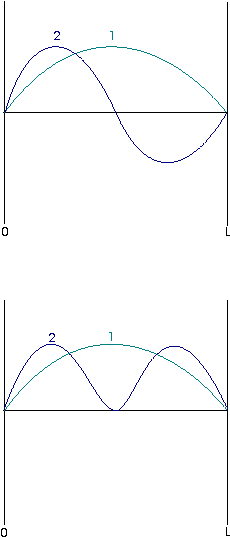
This is an example of the correspondence principle, which states that at high quantum numbers, quantum mechanical results reduce to classical mechanical ones.The probability distribution is decidedly nonuniform at low quantum numbers, but as n increases, the probability distribution does become more uniform. The distribution at high quantum numbers is consistent with the classical result that a particle moving between the two walls should, on average, spend equal amounts of time at all positions within the box.